Probability

Basics

• Probability means possibility. It is a branch of mathematics that deals with the occurrence of a random event.

• The value of probability of an event is expressed from zero to one.

• Probability has been introduced in Maths to predict how likely events are to happen.

• The meaning of probability is basically the extent to which something is likely to happen.

• This is the basic probability theory, which is also used in the probability distribution, where you will learn the possibility of outcomes for a random experiment.

• To find the probability of a single event to occur, first, we should know the total number of possible outcomes.

• Probability is a measure of the likelihood of an event to occur. Many events cannot be predicted with total certainty. We can predict only the chance of an event to occur i.e. how likely they are to happen, using it.

• Probability can range in from 0 to 1, where 0 means the event to be an impossible one and 1 indicates a certain event.

• The probability of all the events in a sample space adds up to 1.

• For example, when we toss a coin, either we get Head OR Tail, only two possible outcomes are possible (H, T). But if we toss two coins in the air, there could be three possibilities of events to occur, such as both the coins show heads or both show tails or one shows heads and one tail, i.e. (H, H), (H, T),(T, T).

• The probability formula is defined as the probability of an event to happen is equal to the ratio of the number of favourable outcomes and the total number of outcomes.

• Probability of event to happen P(E)=(Number of favourable outcomes)/(Total number of outcomes)

Basic concept on drawing a card:

• In a pack or deck of 52 playing cards, they are divided into 4 suits of 13 cards each i.e. spades ♠ hearts ♥, diamonds ♦, clubs ♣.
• Cards of Spades and clubs are black cards.
• Cards of hearts and diamonds are red cards.
• The card in each suit are ace, king, queen, jack/knaves, 10, 9, 8, 7, 6, 5, 4, 3 and 2.
• King, Queen and Jack (or Knaves) are face cards. So, there are 12 face cards in the deck of 52 playing cards.

Check out our CSAT Course

Practice Questions

Q1. A card is drawn from a well shuffled pack of 52 cards. Find the probability of:
(i) ‘2’ of spades
(ii) a jack
(iii) a king of red colour
(iv) a card of diamond
(v) a king or a queen
(vi) a non-face cards
(vii) a black face card
(viii) a black card
(ix) a non-ace
(x) non-face card of black colour
(xi) neither a spade nor a jack
(xii) neither a heart nor a red king

Q2. A card is drawn at random from a well-shuffled pack of cards numbered 1 to 20. Find the probability of
(i) getting a number less than 7
(ii) getting a number divisible by 3.

Q3. A card is drawn at random from a pack of 52 playing cards. Find the probability that the card drawn is
(i) a king
(ii) neither a queen nor a jack.

Q4. Three digits are chosen at random from 1, 2, 3, 4, 5, 6, 7, 8 and 9 without repeating any digit. What is the probability that the product is odd?
a. 1/2
b. 3/5
c. 7/5
d. 1/6

Q5. What is the probability of getting an even number in the single throw of a dice?
a. 1/2
b. 3/4
c. 7/5
d. 4/7

Q6. A complete cycle of a traffic light takes 60 seconds. During each cycle the light is green for 25 seconds, yellow for 5 seconds and red for 30 seconds. At a randomly chosen time, the probability that the light will not be green, is?

Q7. Ten identical particles are moving randomly inside a closed box. what is the probability that at any given point of time all the ten particles will be lying in the same half of the box?

Q8. Two coins are tossed 500 times, and we get:
Two heads: 105 times
One head: 275 times
No head: 120 times
Find the probability of each event to occur.

Q9. A tyre manufacturing company kept a record of the distance covered before a tyre needed to be replaced. The table shows the results of 1000 cases.

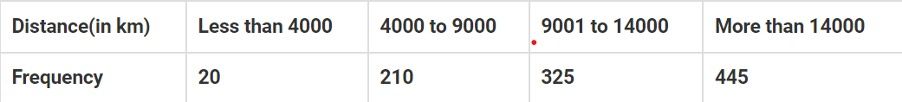
If a tyre is bought from this company, what is the probability that:
(i) it has to be substituted before 4000 km is covered?
(ii) it will last more than 9000 km?
(iii) it has to be replaced after 4000 km and 14000 km is covered by it?

Q10. Two players, Sangeet and Rashmi, play a tennis match. The probability of Sangeet winning the match is 0.62. What is the probability that Rashmi will win the match?

11.A bag contains 20 balls. If there are red, 7 white, and 5 green balls, what is the minimum number of balls a person must pick from the bag to assured of one of each colour?
a. 17
b. 16
c. 13
d. 11

12. A bag contains 15 red balls and 20 black balls. Each ball is numbered either 1 or 2 or 3. 20% of the red balls are numbered 1 and 40% of them are numbered 3. Similarly, among the black balls, 45% are numbered 2 and 30% are numbered 3. A boy picks a ball at random. He wins if the ball is red and numbered 3 or if it is black and numbered 1 or 2. What are the chances of his winning?
a. 1/2
b. 4/7
c. 5/9
d. 12/13

13. Tickets numbered 1 to 20 are mixed up and then a ticket is drawn at random. What is the probability that the ticket drawn has a number which is a multiple of 3 or 5?
a. 1/2
b. 2/5
c. 8/15
d. 9/20

Log-in to access answers for Probability and other topics!

If you have made it so far, well done. You are ahead of the competition.

Best of luck!